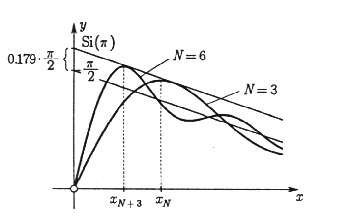
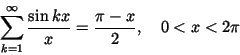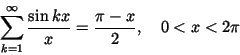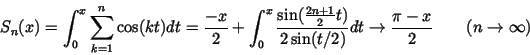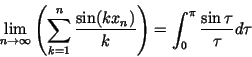The Gibbs Phenomenon

Near a point, where f has a jump discontinuity, the partial sums S_n of a Fourier series exhibit a substantial overshoot near these endpoints, and an increase in n will not diminish the amplitude of the overshoot, although with increasing n the the overshoot occurs over smaller and smaller intervals. This phenomenon is called Gibbs phenomenon. In this section we examine some detail in the behaviour of the partial sums S_n of .

Theorem 6

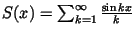The next step is to replace the partial sums S_n with integrals

For 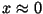we have a typically "overshoot". This will be the next step to show. Let .

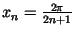Theorem 7 (The Gibbs phenomenon)
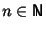
Let and .

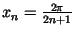
and

Since 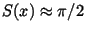for x near 0, we see that an "overshoot" by approximately 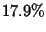is maintained as 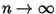(but over smaller and smaller intervals centred at x=0).